Step-by-step explanation:
Given;
We are told that a couple of friends want to produce placemats at a cost of $0.75 per item. Also we are told that one of the two chose to sell at the rate of $10 per item while the other wants to sell at the rate of $65 per item.
Required;
We are required to write an equation for the company's costs.
Step-by-step solution;
The production of the placemats does not have any fixed costs, rather it would cost $0.75 each. taking the number produced to be x, we can derive an equation for the cost of producing x amounts as follows;
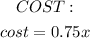
We should also note that the profit would be calculated as shown below;

The revenue generated would be derived as the quantity produced times the price each quantity is sold. Hence, if x number of placemats is produced then, the revenue will be;
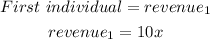
Therefore, for the first individual;
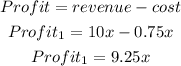
For the second individual;
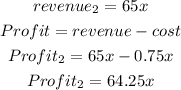
We now have two different profits achievable depending on the price option chosen.
If the friends sell their placemats at school, it would be wiser to choose price option 1 (that is $10.00 each). The profit achievable here is smaller however, it would more realistic to sell to students at the rate of $10 each because a price as high as $65 per placemat would attract very low (if any) buyers on a school campus.
On the other hand if selling at a fine arts festival, it would be wiser to choose price option 2 (that is $65.00 each). The profit achievable at this price is much higher but is achievable because a fine arts festival attracts a high number of affluent fine arts lovers who would be wiling to pay more for an artwork as long as they like it. That means most art lovers at an art festival would prefer one that costs higher believing its of better quality.