We can identify a, h and k by looking at the general formula of a parabola in vertex form and comparing with the equations that you got:

Where a is the stretches of the parabola, h is the x coordinate of the vertex and k is the y-coordinate of the vertex.
In te equation:
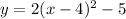
h=4, since is the value that we are subtracting from x inside the parentheses, a=2, since it's the number that is multiplying by (x-h)^2 and k= -5, since this is the value that we add to the first term.
then a=2, h=4 and k= -5
In the equation:
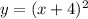
h= -4, so when you subtract -4 from x you get x-(-4)=x+4 inside the parentheses, a=1, since the term (x-h)^2 hasn't a coefficient multiplying by it, and k= 0, since we are adding nothing to the first term.
then a=1, h= -4 and k= 0
In the equation:

h=6, since is the value that we are subtracting from x inside the parentheses, a= -1, since it's the number that is multiplying by (x-h)^2 and k= 3, since this is the value that we add to the first term.
then a= -1, h=6 and k= 3