Step 1:
Concept
Two lines are parallel if their slopes are equal.
Two lines are perpendicular if product of their slopes = -1
Step 2:
Find the slope of each line by writing each equation in the form of y = mx + c.
m = slope and c = intercept on y-axis
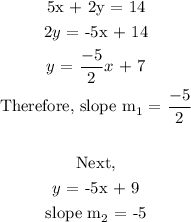
Final answer

Option C neither