Answers:
1. One solution
2. Infinite solutions
Step-by-step explanation:
For the first system of equations, we have:
y = 2x - 10
y + 3x = 5
So, we can use the first equation and replace y by (2x-10) on the second equation and solve for x, so:
y + 3x = 5
2x - 10 + 3x = 5
5x - 10 = 5
5x - 10 + 10 = 5 + 10
5x = 15
5x/5 = 15/5
x = 3
Then, we can replace x by 3 on the first equation and calculate y, so:
y = 2x - 10
y = 2(3) - 10
y = 6 - 10
y = -4
Therefore, the first system has one solution and the solution is (3, -4)
On the other hand, for the second system, we have:
2x + y = 2
6x + 3y = 6
So, we can divide both sides of the second equation by 3 and get an equivalent equation as:
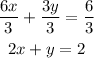
Since the second and first equations are equivalent, the system has infinite solutions.