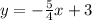The slope-intercep form is

where m is the slope and b the y-intercept. We can find m by means of the following formula

where these values come from the given points,

Then, by substituting these values into the slope formula, we get

which gives

Then, our line equation has the form
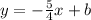
Now, we can find b by subtituting one of the given point into our last equation, that is, If we substitute point (0,3) we obtain

Then, the line equation in slope-intercept form is