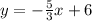Question

(6,-4),(9,-9) in slope intercept form please and thank you

Answer 1

The slope-intercept of a line is

`y=mx+b`

where m is the slope and b is the y-intercept.

Now, the slope m is defined as

`m=\frac{\text{rise}}{\text{run}}=(y_1-y_2)/(x_1-x_2)`

where (x_1, y_1) and (x_2, y_2) are any two points on a line.

Now, in our case the two points are (6,-4) and (9,-9); therefore, the slope is

`m=(-9-(-4))/(9-6)=-(5)/(3)`

With, the value of b in hand the equation of the line becomes

`y=-(5)/(3)x+b`

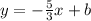
and now we just need to find b. To do this we use any one of the two points and substitute into the above equation and then solve for b. For our purposes, let us just random choose (6,-4) (point (9,-9) would have worked just as well) and substitute into the equation to get:

`-4=-(5)/(3)(6)+b`
`-4=-10+b`

`\rightarrow b=6.`

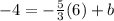
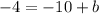
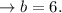
Hence, the equation of the line in slope-intercept form is

`y=-(5)/(3)x+6`