Question

Find the two consecutive numbers whose product is 30.3 and 105 and 615 and 230 and 1

Answer 1

Answer;

5 and 6

Explanations

Let the two consecutive numbers be x and x+1. If the product of the numbers is 30, hence;

`x(x+1)=30`

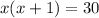
Expand the expression

`\begin{gathered} x^2+x=30 \\ x^2+x-30=0 \end{gathered}`

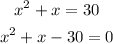
Factorize

`\begin{gathered} x^2+6x-5x-30=0 \\ x(x+6)-5(x+6)=0 \\ (x-5)(x+6)=0 \\ x=5\text{ }and-6 \end{gathered}`

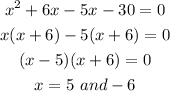
First number = 5

Second number = x+ 1 = 5+ 1

Second number = 6

Hence the two consecutive numbers whose product is 30 are 5 and 6