Answer:
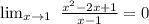
Explanation:
We can have an estimate for a limit by evaluating the expression at points that are close to where the limit is, then for:

We can assume, the following close values for the limit.
0.9,0.99,0.999,0.9999
1.1,1.01,1.001,1.0001
Plug them, into the function and solve to investigate the behavior.
As we can see on the image above, the behavior is getting closer to 0, then the limit of the function as it approaches 1 is 0.