Question

Kevin decides to mix grades of gasoline in his truck. He puts in 5 gallons of regular and 10 gallons of premium for a total cost of $61.30. If premium gasoline costs $0.22 more per gallon than regular, what was the price of each grade of gasoline?

Answer 1

Let be:

• r: The price of a grade of regular gasoline.

,

• p: The price of a grade of premium gasoline.

Then, from the word problem, we know that:

`\begin{gathered} p=0.22+r\Rightarrow\text{ Equation 1} \\ 5r+10p=61.30\Rightarrow\text{ Equation 2} \end{gathered}`

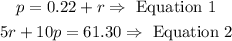
Thus, we can write the following equation:

`5r+10(0.22+r)=61.30`

Now, we can solve the above equation for r:

`\begin{gathered} 5r+10(0.22+r)=61.30 \\ \text{ Apply the distributive property} \\ 5r+10\cdot0.22+10\cdot r=61.30 \\ 5r+2.2+10r=61.30 \\ \text{ Add similar terms} \\ 2.2+15r=61.30 \\ \text{ Subtract }2.2\text{ from both sides of the equation} \\ 2.2+15r-2.2=61.30-2.2 \\ 15r=59.1 \\ \text{ Divide by 15 from both sides of the equation} \\ (15r)/(15)=(59.1)/(15) \\ r=3.94 \end{gathered}`

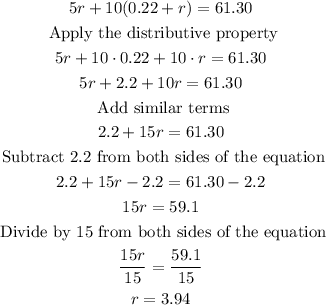
Finally, we replace the value of r in Equation 1, and we solve it for p:

`\begin{gathered} p=0.22+r \\ p=0.22+3.94 \\ p=4.16 \end{gathered}`

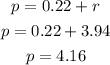
Therefore, the price of a grade of regular gasoline is $3.94, and of a grade of premium gasoline is $4.16.