1. Use the given rule to find the coordinates of A'B'C':
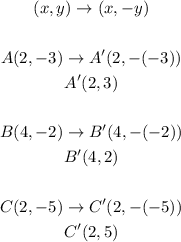
2. Graph:
3. Description:
The given transformation is a reflection over x-axis. Points in the image have the same x coordinate than the points in the preimage, but the y coordiante changes of sign (it changes from one side to another of the x-axis)
Then, triangle ABC is refelcted over x-axis to get triangle A'B'C'