Question

I really need help with part B and C. A is already done so I'll include A so you can use it to help you answer the rest Answer of part A: 2.2 times more intense. The intensity of the Seattle roar is in the picture

Answer 1

B. 139.6 dB

C. 100 W/m²

Step-by-step explanation

B. From the first part, we have that the intensity of the Seattle roar was 45.7088 W/m². Now, the fans in Kansas City want to create a roar twice as intense,

`I=2\cdot45.7088W/m^2=91.4176W/m^2`

To find how many decibels this roar needs to be, we have to replace I with this value in the given equation,

`L=10\log \mleft((91.4176)/(10^(-12))\mright)\approx10\cdot13.96=139.6dB`

The fans in Kansas City have to roar at 139.6 dB.

C. Now, their roar was 140 dB and we have to find the intensity. In the given equation, replace L with 140,

`140=10\log \mleft((I)/(I_o)\mright)`

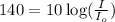
And solve for I. Divide both sides by 10,

`(140)/(10)=\log \mleft((I)/(I_o)\mright)`

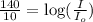
Rise 10 to the exponent of each side. By the rule of the exponent of the base of a logarithm, the result on the right side of the equation is what is in the parenthesis,

`\begin{gathered} 10^{(140)/(10)}=10^{\log ((I)/(I_o))} \\ \\ 10^{(140)/(10)}=(I)/(I_o) \end{gathered}`

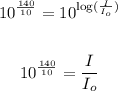
Finally, multiply both sides by I₀,

`I=I_o\cdot10^{(140)/(10)}`

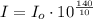
Replace the value of Io given and solve,

`I=10^(-12)\cdot10^{(140)/(10)}=100W/m^2`

Hence, the intensity of the roar of the fans in Kansas City was 100 W/m².