We are given the following system of equations:

We can simplify equation (2) by dividing both sides by 2, we get:

Now, we substitute the value of "x" from equation (1) into equation (2):
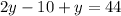
Now, we add like terms:
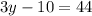
Now, we add 10 to both sides:
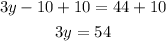
Now, we divide both sides by 3:
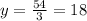
Now, we substitute this value of "y" in equation (1):
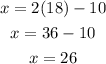
Therefore, the length of the stamp is 26 mm and its width is 18mm.
We notice that:

This is due to the fact that:
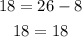
Therefore, the width of the stamp is 8mm less than the length.