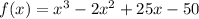Given
The zeros are given 2, 5 i and -5 i.
Step-by-step explanation
To determine the polynomial function ,
Use the general expression for the polynomial.
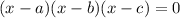
Substitute the values,
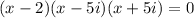
Use the identity to simplify the expression
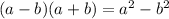
Then the expression becomes.
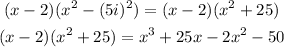
Answer
The answer is