Question

b. A weight of pounds corresponds to the 5th percentile for newborn hippos.(Round to the nearest integer as needed.)

Answer 1

`z=(x-\mu)/(\sigma)`

Solve x in the formula above:

`\begin{gathered} z\sigma=x-\mu \\ \\ x=z\sigma+\mu \end{gathered}`

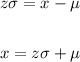
To find the value (x) corresponding to the 5th percentile:

1. Use a z-table to find the z corresponding to 5th percentile (0.05)

2. Evaluate the formula of x with the next data:

`\begin{gathered} z=-1.64 \\ \mu=84 \\ \sigma=12 \end{gathered}`
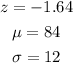
`\begin{gathered} x=(-1.64)(12)+84 \\ x=-19.68+84 \\ x=64.32 \end{gathered}`Then, A weight of 64 pounds corresponds to the 5th percentile for newborn hippos