We can draw the following picture:
From the figure, we can see that triangles ABC and EDC are similar.
Reasons:
By means of AAA (angle-angle-angle) theorem those triangles are similar, that is
they both triangles have the same angles (red, blue, green).
Since both triangles are similar the following ratio must be preserved:
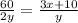
Now, from this ratio, we must find x.
If we move 2y to the right hand side, we get

so we can eliminate y in both, the denominator and numerator. Then, we obtain
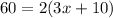
which is equal to

If we move 20 to the left hand side as -20, we get
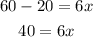
then, we have
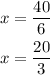
Therefore, x is equal to 20/3.