Answer:
4.16 x 10^6 seconds
Step-by-step explanation:
First, we need to find the distance of Jupiter from the Sun in meters. So, we know that 1 mile has 1609.34 meters. So, 7.8 x 10^11 miles are equivalent to:

Then, to calculate the number of seconds that the sunlight needs to reach Jupiter, we need to divide the distance from the Sun to Jupiter by the speed of the light as:
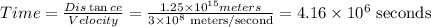
Therefore, the sunlight takes 4.16 x 10^6 seconds to reach Jupiter