Question

what is the area of the cross section of a cast iron pipe if the inside diameter is 12in and the thickness of the metal is 1/2 in

Answer 1

Picture the situation: draw a circle of diameter 12 in and another with the same center with radius 1/2 more than the previous circle:

The cross-section area will be the difference from the area of the bigger circle minus the area of the smaller circle.

Since the radius of a circle is half its diameter, then the radius of the inner circle is 6in.

Since the radius of the exterior circle is 1/2 in bigger than the radius of the inner circle, then its radius is 6.5 in.

Calculate both areas and then substract them:

`\begin{gathered} A_1=\pi\cdot r^2_1 \\ =\pi\cdot(6in)^2 \\ =36\pi in^2 \end{gathered}`
`\begin{gathered} A_2=\pi\cdot r^2_2 \\ =\pi\cdot(6.5in)^2 \\ =42.25\pi in^2 \end{gathered}`

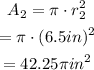
The area of the cross section is:

`\begin{gathered} A=A_2-A_1 \\ =42.25\pi in^2-36\pi in^2 \\ =6.25\pi in^2 \\ =19.63495408\ldots in^2 \\ \approx19.6in^2 \end{gathered}`

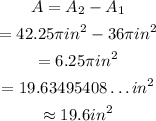
Therefore, the area of the cross section is approximately 19.6 squared inches.