Question

Help me answer this question please ( I got 2 and 1 and it was wrong)

Answer 1

Step-by-step explanation:

A critical point is a point where the function is undefined or its derivative is zero or undefined.

For this function, it is always defined in the given domain. We have to see the derivative:

`(dg(x))/(dx)=g^(\prime)(x)=-3\sin x`

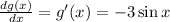
It is also always defined in the domain, but it has a zero:

`\begin{gathered} -3\sin x=0 \\ \sin x=0 \\ x=n\pi\text{ , }n\in\Z \end{gathered}`

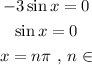
n is ...-3, -2, -1, 0, 1, 2, 3, ...

So there's only one critical point in the domain, and that's x = 0.

Answer:

There's only 1 critical point in the domain: 0