Question

Using the net of each solid shape, find its surface area.

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Find the area of J

`\begin{gathered} J\text{ is a triangle} \\ Area\text{ of triangle}=(1)/(2)* b* h \\ Area\text{ of triangle}=(1)/(2)*6*8=3*8=24ft^2 \end{gathered}`

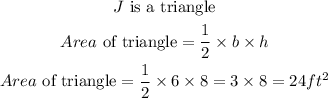
STEP 2: Find the area of K

`\begin{gathered} K\text{ is a rectangle} \\ Area\text{ of rectangle}=length* width \\ Area=8ft*9ft=72ft^2 \end{gathered}`

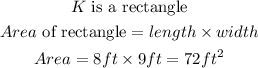
STEP 3: Find the area of L

`\begin{gathered} L\text{ is a rectangle} \\ Area\text{ of rectangle}=length* width \\ Area=9ft*6ft=54ft^2 \end{gathered}`

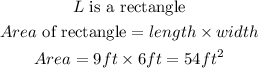
STEP 4: Find the area of M

`\begin{gathered} M\text{ is a Rectangle} \\ Area\text{ of rectangle}=length* width \\ Area=9ft*10ft=90ft^2 \end{gathered}`

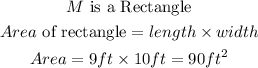
STEP 4: Find the area of N

`\begin{gathered} N\text{ is a triangle with same dimensions as J} \\ Therefore,\text{ the area is the same as J} \\ Area=24ft^2 \end{gathered}`

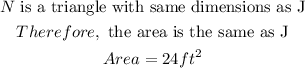
STEP 5: Find the surface area

To determine the surface area of a solid, we take the sum of the area of all the surfaces of a 3-dimensional solid object.

`24ft^2+72ft^2+54ft^2+90ft^2+24ft^2=264ft^2`