Question

The graph below shows the different paths taken by the human cannonball when his velocity out of the cannon is 50 miles/hour, and his cannon is inclined at varying angles.a. If his landing net is placed 104 feet from the cannon, at what angle should the cannon be inclined so that he lands in the net. Only answer 1 of the possibilities ____ b. Approximately where do you think he would land if the cannon was inclined at 45°?____feetc. If the cannon was inclined at 45°, approximately what height do you think he would attain?____feetd. Do you think there is another angle for which he would travel the same distance he travels at 80°. Give an estimate of that angle.____

Answer 1

Step-by-step explanation

In this problem, we have the graph of the trajectory of a cannonball shot with different angles.

(a) If we place a landing net at a distance of 104 ft from the cannon, we want the cannonball to land approximately at the point marked in yellow in the following graph:

From the graph, we see that the trajectories with an angle of 20° or 70° will leave the cannonball at a distance of 104 ft.

(b) If the cannonball was inclined at 45°, it will follow a tractory very near to the ones with angles of 40° and 50°, so the cannonball will land at a distance of approximately 170 ft.

(c) If the cannonball was inclined at 45°, it will follow a trajectory that lies in the middle of the ones with angles of 40° and 50°, so its maximum height will be:

`h(45\degree)=(h(50\degree)+h(40\degree))/(2)=(50ft+35ft)/(2)=42.5ft.`

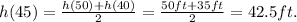
(d) Another angle that can make travelling the cannonball a distance equal to the angle of 80° is 10°.

Answer

(a) 20°

(b) 170

(c) 42.5

(d) Yes, 10°