Given the information on the problem, we can write the following function:
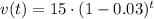
where v(t) denotes the weight of the element at time t.
Then, to find the time it will take to the element to weight only 3 grams, we have to solve v(t) = 3:
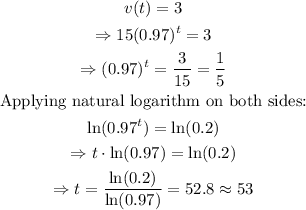
therefore, it will take approximately 53 years for the element to weight 3 grams