Question

I need to know a lot, I need help

Answer 1

we have

the system of inequalities

`\begin{gathered} y\text{ < 6x-5} \\ y\ge-2x \end{gathered}`

Remember that

If a ordered pair is a solution of the system of inequalities, then the ordered pair must satisfy both inequalities

so

we have the ordered pair

(1,2)

Verify inequality 1

x=1, y=2

`\begin{gathered} 2\text{ < 6(1)-5} \\ 2\text{ <1 ---}\longrightarrow\text{ is not true} \end{gathered}`

therefore

The ordered pair is not a solution of the system of inequalities

we have the ordered pair

(1,-4)

x=1, y=-4

Verify inequality 1

`\begin{gathered} -4\text{ < 6(1)-5} \\ -4<\text{ 1 ---}\longrightarrow\text{ is true} \end{gathered}`

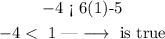
Verify inequality 2

`\begin{gathered} -4\text{ }\ge-2(1) \\ -4\ge-2\text{ ---}\longrightarrow\text{ is not true} \end{gathered}`

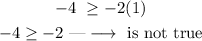
therefore

teh ordered pair is not a solution for the system of inequalities

we have the ordered pair

(-2,6)

Verify inequality 1

x=-2, y=6

`\begin{gathered} 6<6(-2)+5 \\ 6<-7\text{ ----}\longrightarrow\text{ is not true} \end{gathered}`

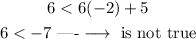
therefore

the ordered pair is not a solution of the system of inequalities

we have

(-5,10)

x=-5, y=10

Verify inequality 1

`\begin{gathered} 10<\text{ 6(-5)-5} \\ 10<\text{ -35 ---}\longrightarrow\text{ is not true} \end{gathered}`

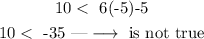
the ordered pair is not a solution of the system of inequalities