ANSWER
![x=(3)/(10)+\frac{\sqrt[]{91}}{10}i;x=(3)/(10)-\frac{\sqrt[]{91}}{10}i](https://img.qammunity.org/2023/formulas/mathematics/college/mxdge5193eiajv503degwp342yvgso171d.png)
Step-by-step explanation
To solve the equation given, we have to apply the quadratic formula:
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
where a = coefficient of x²
b = coefficient of x
c = constant term
From the equation, we have that:
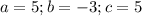
Therefore, solving for x, we have:
![\begin{gathered} x=\frac{-(-3)\pm\sqrt[]{(-3)^2-4(5)(5)}}{2(5)} \\ x=\frac{3\pm\sqrt[]{9-100}}{10}=\frac{3\pm\sqrt[]{-91}}{10}=\frac{3+\sqrt[]{-1\cdot91}_{}}{10} \\ \Rightarrow x=\frac{3\pm\sqrt[]{91}i}{10} \\ \Rightarrow x=(3)/(10)+\frac{\sqrt[]{91}}{10}i;x=(3)/(10)-\frac{\sqrt[]{91}}{10}i \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/9w15pigljibcka5nz3fbjrfdxd5wnc4qxn.png)
That is the solution to the equation.