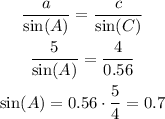First let's draw this triangle:
Now, let's find the cosine of C using the law of cosines:
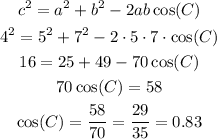
Now, in order to find the sin(A) and tan(C), let's first find the sin(C) using the property:
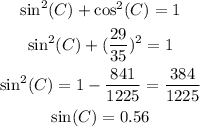
We can find the tan(C) using the relation:
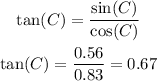
Finally, we can find sin(A) using the law of sines: