An art teacher has a box of markers.
She keeps half of the markers in the box and gives 1/3 of the other half to group A.
Group A gets,
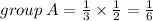
So, group A gets 1/6 markers.
The remaining markers were shared equally among 8 students in group B.
Remaining markers means
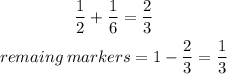
So, group B gets

Therefore, each student of group B gets 1/24 of the whole box