Question

A school is painting its logo in the shape of a triangle in the middle of its sports field. The school wants the height of the triangle to be 4 feet. The area of thelogo must be at least 10 square feet. (The logo has to be seen from all the seats.) Write an inequality that describes the possible base lengths (in feet) of thetriangle.Use b for the base length of the triangular logo.

Answer 1

We know that

• The height of the triangle is 4 feet.

,

• The area must be at least 10 square feet.

Using the given information, we express the following inequality. Remember that the area of a triangle the semi-product of the base and height.

`(b\cdot4)/(2)\ge10`

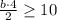
Then, we solve for b.

`\begin{gathered} 2b\ge10 \\ b\ge(10)/(2) \\ b\ge5 \end{gathered}`

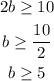
The possible base lengths are all numbers greater than or equal to 5.