Answer:
3744 cm³
Step-by-step explanation:
The given prism has a trapezium as its cross-section.
For any prism:
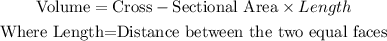
Cross-Sectional Area
![\begin{gathered} \text{Area of a trapezium=}(1)/(2)(a+b)h \\ a=23\operatorname{cm} \\ b=16\operatorname{cm} \\ h=24\operatorname{cm} \end{gathered}]()
Thus:
![\begin{gathered} \text{A=}(1)/(2)(23+16)24 \\ =(1)/(2)(39)24 \\ =468\operatorname{cm}^2 \end{gathered}]()
The distance between the two trapezoidal faces is 8cm.
Therefore, the length of the prism = 8cm.
![\begin{gathered} \text{Volume}=468*8 \\ =3744\operatorname{cm}^3 \end{gathered}]()
The volume of the prism is 3,744 cubic centimetres.