After sketching the rectangular box, notice that the black line is the diagonal.
Along with the red side and the green diagonal of one of the faces, it is the hypotenuse of a right triangle.
Let D be the diagonal of the rectangular box.
Let d be the diagonal of one of the faces of the box, with sides of lengths a and b.
Let c be the length of the remaining side of the box, which makes a right triangle with the diagonals D and d.
Applying the Pythagorean Theorem to the sides D, d, and c, it is known that:
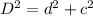
Applying the same theorem to the sides d, a and b, it is known that:

Substitute the expression for d^2 given by the last equation on the first equation:
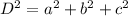
Take the positive square root of both sides of the equation:
![D=\sqrt[]{a^2+b^2+c^2}](https://img.qammunity.org/2023/formulas/mathematics/college/bw1xlkzy54vvrxpfrtc9x27hczf1297glp.png)
Substitute a=12, b=12 and c=18 into the equation for D:
![D=\sqrt[]{12^2+12^2+18^2}](https://img.qammunity.org/2023/formulas/mathematics/college/if81yio1eesorjijxo6363127xvsjxeqcf.png)
Evaluate the expression for D:
![D=6\cdot\sqrt[]{17}\approx24.74](https://img.qammunity.org/2023/formulas/mathematics/college/nlmrk0vuo977hvhiofj2qwlv9gg83hcc8l.png)
Therefore, the diagonal of the box has a length of:
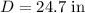
Aknowledgement: All the diagonals of a rectangular box have the same length.