Answer:
No Solution
Step-by-step explanation:
Given the system of equations:
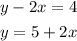
First, graph each equation using the x and y-intercepts.
Equation 1
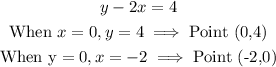
Join the points (0,4) and (-2,0) as done below:
Equation 2
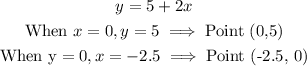
Join the points (0,5) and (-2.5, 0) as done below:
We observe that the two lines are parallel.
Therefore, the system of equations has No Solution.