Since the population grows following a linear model, this means that we can write the relationship between the population and the time in a linear equation.
The standard form of a linear equation is:

Where:
m = slope
b = y-intercept
Also, given two points, P and Q, we can find the slope of the line that connects them by:
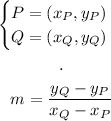
The problem tells us that at week 0, the population is 3, and at week 8 the population is 51. Those are two points that we can call:
P = (0, 3)
Q = (8, 51)
Now, we can calculate the slope:
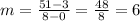
And since the y-intercept is the value of y when x = 0, the y-intercept is the population at week 0, b = 3
Then:

Is the explicit formula for the beetle population after n weeks.
Now, to find after how many weeks the beetle population will be 165, we substitute in the equation P = 165:
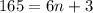
And solve:
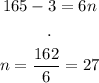
Thus, after 27 weeks the population will be 165.