First, lets write the angles in decimal form:
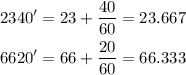
Now, notice that the sum of these two angles gives us a right angle:
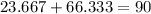
Next, we have to find out how many miles they traveled after the 4 hours, then, we have the following:
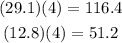
therefore, the triangle of the picture is a right triangle with the following meausres:
using the pythagorean theorem, we get the following:
![\begin{gathered} c^2=(116.4)^2+(51.2)^2=13548.96+2621.44 \\ \Rightarrow c^2=16170.4 \\ \Rightarrow c=\sqrt[]{16170.4}=127.2 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/xchoorvsy0q5faxxagago36kcmtar78e8v.png)
therefore, after 4 hours, the boats are 127.2 miles apart