Answer:
Step-by-step explanation:
a) Here, we want to find the equation of the directrix of the parabola.
We have that as follows:
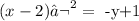
We compare the above with the following:
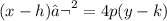
what we have here will be:
h = 2, k = -1 and p = -1/4
Directrix:
y = k-p
y = -1 + 1/4 = -3/4
b) Vertex
We have the vertex as (h,k)

c) Focus