The given functions are:
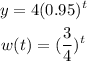
For the first one, we can write 0.95 as:
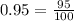
Then, we have the function:
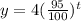
Applying the power of quotient property we have:
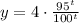
Thus, as 100 is greater than 95, the denominator will always be greater than the numerator, which means the result of the division will be closer to 0 as t grows. In this case we have then an exponential decay because the function is decaying.
For the second function, applying the same property of powers we have:
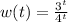
Then, we have the same situation, as the denominator is bigger than the numerator, it will grow faster than the numerator, and the result of the division will be closer to zero as t gets bigger. In this case, we also have an exponential decay.
The percent rate of change in exponential decay is given by:
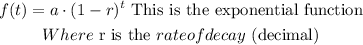
Then, for the first function:
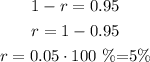
The percent rate of change for y=4(0.95)^t is 5%
For the second function:
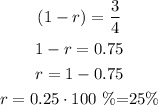
The percent rate of change for w(t)=(3/4)^t is 25%.