Question

If A is at 2,2 and B at 3,4 is the midpoint of segment AC ,what are the coordinates of C

Answer 1

Answer:

The coordinates of C are (4, 6)

Explanations:

Coordinates of point A = (2, 2)

Coordinates of point B = (3, 4)

B is the midpoint of line AC

The coordinates of the midpoint of a line are given by the formula:

`\begin{gathered} a\text{ = }(x_(1+)x_2)/(2) \\ b\text{ = }(y_1+y_2)/(2) \\ \text{Where a = 3, b =4},x_1=2,y_1=\text{ 2} \\ \text{Substitute these coordinates into the fourmulae above:} \\ 3\text{ = }\frac{2+_{}x_2}{2} \\ 3(2)=2+x_2 \\ 6\text{ = 2 + }x_2 \\ x_2=\text{ 6 - 2} \\ x_2=\text{ 4} \\ 4\text{ = }\frac{2+_{}y_2_{}}{2} \\ 4(2)=2+y_2 \\ 8=2+y_2 \\ y_2=\text{ 8 - 2} \\ y_2=\text{ 6} \end{gathered}`

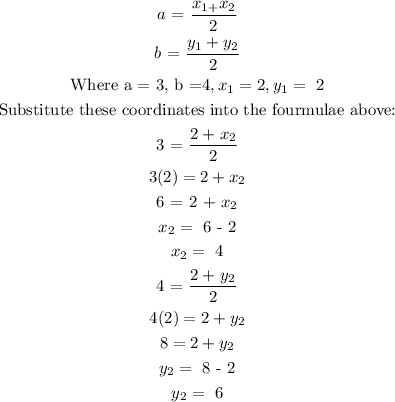
Therefore the coordinates of C are (4, 6)