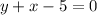Question

Find the equation of the line through (-1,6) and has x-intercept of 5. Write your answer in general form.

Answer 1

We have two points, the first one is (-1,6), and the second is the x-intercept, the point (5,0).

First, find the slope using the next formula:

`m=(y_2-y_1)/(x_2-x_1)`

Replace these values:

`m=(0-6)/(5-(-1))=(-6)/(6)=-1`

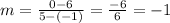
Then, m=-1

To find the general form use the next formula:

`y-6=-1(x-(-1)`
`y-6=-x-1`

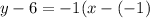
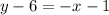
Equal the whole expression to zero.

Add both sides by x:

`y-6+x=-x+x-1`
`y-6+x=-1`

Add both sides by 1:

`y+x-6+1=-1+1`

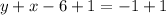
Then, the general form of the line equation is:

`y+x-5=0`