Given the polynomial:
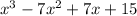
You can factorize it as follow:
1. Rewrite the term with exponent 2 in this form:
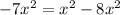
2. Rewrite the x-term in this form:
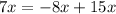
3. Rewrite the expression:
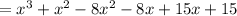
4. Make three groups of two terms each using parentheses:
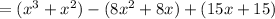
5. Identify the Greatest Common Factor (GCF) of each group (the largest factor that all the terms in the group have in common):
- For:
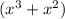
The Greatest Common Factor is:
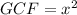
- For:
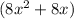
The Greatest Common Factor is:
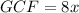
- And for:
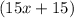
It is:
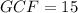
6. Factor the GCF of each group out:
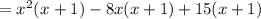
7. Notice that each expression is common in all the terms:
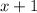
Then, you can factor it out:
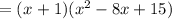
8. In order to factor the Quadratic Polynomial in the second parentheses, you can find two numbers whose Sum is -8 and whose Product is 15. These are -3 and -5. Then, you get:
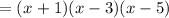
Hence, the answer is: Option D.