Answer:
9765625
Step-by-step explanation:
The nth term of a geometric sequence can be calculated using the following
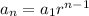
Where a1 is the first term and r is the common ratio. The value of a1 is 1 and the value of r can be calculated as the ratio between two consecutive numbers, so
5/1 = 5
25/5 = 5
125/25 = 5
625/125 = 5
Therefore, r = 5 and a1 = 1. Replacing this values, we get:
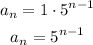
Finally, we can find the 11th term replacing n by 11, so
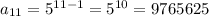
Therefore, the 11th term of the sequence us 9765625