Question

The table shows the classification of students in a school

Answer 1

Given:

Given a table showing the classification of students

Required: Probability

Step-by-step explanation:

i) From the table, the total number of students in the school

`\begin{gathered} =45+40+60+55+50+35+65+50+70+55+45+30 \\ =600 \end{gathered}`

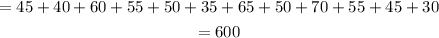
a) Number of boys in the school

`\begin{gathered} =45+60+50+65+70+45 \\ =335 \end{gathered}`

Probability of selecting a boy from the school

`\begin{gathered} =(335)/(600) \\ =(67)/(120) \end{gathered}`

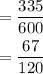
(b) Number of girls in the school

`\begin{gathered} =40+35+55+55+50+30 \\ =265 \end{gathered}`

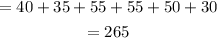
Probability of selecting a girl from the school

`\begin{gathered} =(265)/(600) \\ =(53)/(120) \end{gathered}`

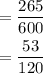
ii)

a) Number of boarders in SS1

`\begin{gathered} =60+55 \\ =115 \end{gathered}`

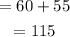
Probability of selecting a boarder in SS1

`\begin{gathered} =(115)/(600) \\ =(23)/(120) \end{gathered}`

b) Number of girls in boarding

`\begin{gathered} =55+50+30 \\ =135 \end{gathered}`

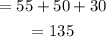
Probability of selecting a boarder that is a girl

`\begin{gathered} =(135)/(600) \\ =(9)/(40) \end{gathered}`

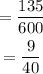
Final Answer:

i) a) Probability of selecting a boy from the school = 67/120

b) Probability of selecting a girl from the school = 53/120

ii) a) Probability of selecting a boarder in SS1 = 23/120

b) Probability of selecting a boarder that is a girl = 9/40