We must compute the area of the shaded region of the rectangle. We see that the area of the shaded region is equal to the area of the rectangle minus the area of the blank square:

Where:
• A = area of the shaded region that surrounds the square,
,
• A_R = area of the rectangle,
,
• A_S = area of the blank square.
1) The dimensions of the rectangle are:
• l = length = 14 in,
,
• w = width = 11 in.
The area of the rectangle is given by:
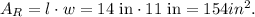
2) The dimensions of the square are:
• a = side = 9 in.
The area of the square is given by:
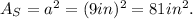
3) Replacing the values obtained for A_R and A_s in the formula of the beginning, we get:
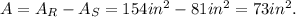
Answer
The area of the region inside the rectangle that surrounds the square (the shaded region) is 73in².