Question

Please explain this thoroughly so that I can understand. It’d be very much appreciated, thank you.

Answer 1

The first step to solve this problem, is to isolate the "y" variable on the left side. This is done below:

`\begin{gathered} -2x+y=3 \\ y=2x+3 \end{gathered}`

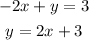
Now we have to find 5 pairs of points that belong to the function, so we can fill the table. We will use values of x and determine the values of y. The x we will use will be:

`\mleft\lbrace-2,-1,0,1,2\mright\rbrace`

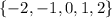
These could be any values, we could have picked at random, but it's good to pick some negative values and some positive ones. Let's find these values:

`\begin{gathered} y=2\cdot(-2)+3 \\ y=-4+3=-1 \end{gathered}`

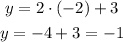
The first point is (-2, -1).

`\begin{gathered} y=2\cdot(-1)+3 \\ y=-2+3=1 \end{gathered}`

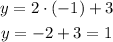
The second point is (-1, 1).

`\begin{gathered} y=2\cdot0+3 \\ y=3 \end{gathered}`

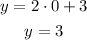
The third point is (0, 3).

`\begin{gathered} y=2\cdot1+3 \\ y=2+3=5 \end{gathered}`

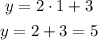
The fourth point is (1, 5).

`\begin{gathered} y=2\cdot2+3 \\ y=4+3 \\ y=7 \end{gathered}`

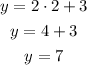
The fith point is (2, 7).

Now we have to graph this equation. For that we need to choose any of the two points that we calculated before and find them on the coordinate plane, then we have to draw a line between them: