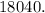Question

The population is decreasing at a rate of 3% per year. If the population is 26,000 today,what will the population be in 12 years? Round your answer to the nearest wholenumber, if necessary.

Answer 1

To answer this question, we will use the following formula for exponential decrease:

`F=I(1-r)^t,`

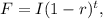
where I is the initial population, F is the final population, r is the rate in decimal form, and t is the time in years.

Substituting r=0.03, I=26,000 and t=12, we get:

`F=26,000(1-0.03)^(12).`

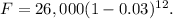
Simplifying the above result, we get:

`F\approx18040.`

Answer:

`18040.`