Given data
9 17 5 5 33 13 1
From the given data
Maximum value Max = 33
Minimum value Min = 1
The mode of the data is the number that appears most or the most occurring number in the set.
The mode of the data = 5
To find the median, arrange the numbers in ascending or descending order of values.
1 5 5 9 13 17 33
The median = 9
The range of the data is the difference between the maximum and the minimum number in the data.
Range = 33 - 1 = 32
Mean is the sum of the numbers divided by the total number of numbers.
![\begin{gathered} \operatorname{mean}\text{ = }\frac{1\text{ + 5 + 5 + 9 + 13 + 17 + 33}}{7} \\ \operatorname{mean}\text{ = }(83)/(7) \\ \operatorname{mean}\text{ = 11.857} \end{gathered}]()
Q1 is the first quartile
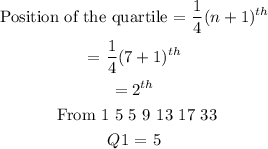
Q3 is the third quartile
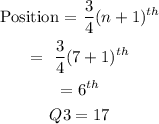
Interquartile range IQR = Q3 - Q1 = 17 - 5 = 12