Question

The equation of the line through (6, 2) and (8,8) is

Answer 1

Given the following points that pass through a graph.

Point 1: (6, 2)

Point 2: (8, 8)

Let's determine the equation of the line.

Step 1: Determine the slope.

`\text{ Slope = m = }\frac{y_2\text{ - y}_1}{x_2\text{ - x}_1}`
`\text{ = }\frac{8\text{ - 2}}{8\text{ - 6}}\text{ = }(6)/(2)`
`\text{ Slope = m = 3}`

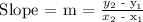
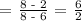
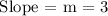
Step 2: Let's determine the y-intercept (b). Plugin m = 3 and x,y = 6, 2 in y = mx + b.

`\text{ y = mx + b}`
`\text{ 2 = \lparen3\rparen\lparen6\rparen + b}`
`\text{ 2 = 18 + b}`
`\text{ b = 2 - 18 = -16}`

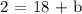
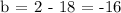
Step 3: Let's complete the equation. Plugin m = 3 and b = -16 in y = mx + b.

`\text{ y = mx + b}`
`\text{ y = \lparen3\rparen x + \lparen-16\rparen}`
`\text{ y = 3x - 16}`

Therefore, the equation of the line is y = 3x - 16