In this problem, we have a binomial probability distribution
where
p=80%=0.80
q=1-p -----> q=0.20 probability of fail
the probability that more than 3 of them are successful is
P(X>3)=P(x=4)+P(X=5)+P(x=6)
step 1
Find out P(X=4)
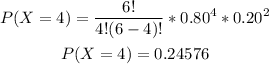
step 2
Find out P(X=5)
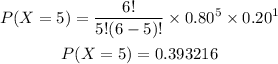
step 3
Find out P(X=6)
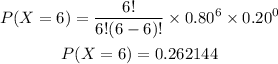
step 4
Adds the probabilities
P(X>3)=0.24576+0.393216+0.262144
P(X>3)=0.90112
therefore
The answer is 0.90