Question

Cam someone help me with this question? my picture got cut off, I can send a better one.

Answer 1

Given:

The side of a parallelogram is, b1 = 6 mm.

The height of a parallelogram is, h1 = 4 mm.

The base of a triangle is, b2 = 8 mm.

The height of a triangle is, h2 = 2 mm.

The objective is to find the area of the figure.

The area of the figure can be calculated as,

`\begin{gathered} A=\text{Area of parallelogram+Area of triangle.} \\ A=(b_1\cdot h_1)+((1)/(2)\cdot b_2\cdot h_2) \end{gathered}`

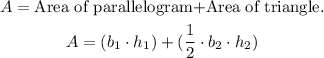
Now, substitute the given values in the above formula.

`\begin{gathered} A=(6\cdot\cdot4)+((1)/(2)\cdot8\cdot2) \\ A=24+8 \\ A=32mm^2 \end{gathered}`

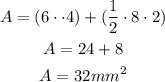
Hence, the area of the figure is 32 square millimeter.