Question

Sequence: a_n=3+6nFind the common difference given: first term is 9

Answer 1

Given:

A sequence is

`a_n=3+6n`

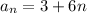
First-term

`a_1=9`

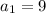
Required:

Find the common difference.

Step-by-step explanation:

Find the terms of the given series.

`\begin{gathered} a_1=9 \\ a_2=3+6(2) \\ a_2=3+12 \\ a_2=15 \end{gathered}`
`\begin{gathered} a_3=3+6(3) \\ a_3=3+18 \\ a_3=21 \end{gathered}`
`\begin{gathered} a_4=3+6(4) \\ a_4=3+24 \\ a_4=27 \end{gathered}`

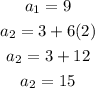
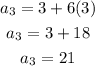
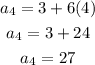
Thus the terms of the given sequence are 9, 15, 21, 27, .......

Now the common difference is:

15 - 9 = 6

21 - 15 = 6

27 - 21 = 6

Final Answer:

The common difference of the given sequence is 6.