Question

Help me wolf this System of equations by the addition/elimination method

Answer 1

Given the system of equations

`\begin{cases}x+y=-3 \\ 3x+4y=-7\end{cases}`

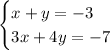
Solve by elimination method as shown below.

Multiply the first equation by 3 and subtract it from the second equation,

`\begin{gathered} 3(x+y)=3(-3) \\ \Rightarrow3x+3y=-9 \end{gathered}`

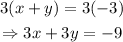
Then,

`\begin{gathered} \Rightarrow(3x+4y)-(3x+3y)=-7-(-9) \\ \Rightarrow y=-7+9=2 \\ \Rightarrow y=2 \end{gathered}`

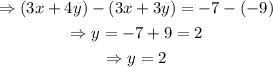
Use the value of y in the first equation,

`\begin{gathered} y=2 \\ \Rightarrow x+2=-3 \\ \Rightarrow x=-5 \end{gathered}`

Therefore, the answers are x=-5 and y=2, (x,y)=(-5,2)