Question

An aircraft factory manufactures airplane engines. The unit cost C (the cost in dollars to make each airplane engine) depends on the number of engines made. If x engines are made, then the unit cost is given by the function C(x) = 1.2x ^ 2 - 504x + 64, 558 . What is the minimum unit cost?Do not round your answer.unit cost?

Answer 1

Step-by-step explanation:

We were given the following information:

`C\left(x\right)=1.2x^2-504x+64558`

We will take the first derivative of the function, we have:

`\begin{gathered} C^(\prime)(x)=2(1.2x^(2-1))-504 \\ C^(\prime)(x)=2.4x-504 \\ \text{We equate the derivative to zero \lparen minimum cost\rparen:} \\ 2.4x-504=0 \\ \text{Add ''504'' to both sides, we have:} \\ 2.4x-504+504=504 \\ 2.4x=504 \\ \text{Divide both sides by ''2.4'', we have:} \\ x=(504)/(2.4) \\ x=210engines \end{gathered}`

We will substitute this into the initial function to obtain the minimum cost. We have:

`\begin{gathered} C(210)=1.2(210)^2-504(210)+64558 \\ C(210)=52920-105840+64558 \\ C(210)=11638 \\ C(210)=\text{\$11,638} \end{gathered}`

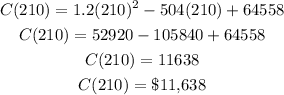
Therefore, the minimum cost is $11,638