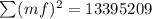we are given a data set and we are asked to determine the following operations:

To determine this, we need to add the products of each of the values of "m" and "f", like this:
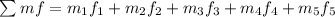
Replacing the values:
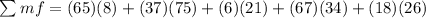
Solving the operation:
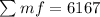
Now we are asked to determine the following operation:
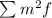
Now we need to add the product of the squares of "m" by "f", like this:

replacing the values:
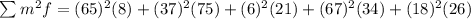
Solving the operations we get:
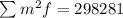
Now we are asked to solve the following operation:
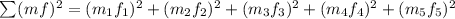
Replacing the values we get:
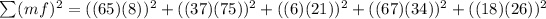
Solving we get: