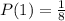Help with number one a-f is all part of number one.Part e is: P(7).

Question

asked Jul 22, 2023 40.7k views

1 Answer

Onel Harrison · Answer 1 · 2023-07-27T03:02:46+0000

Given the spinner that goes from 1 to 8, you can identify that the total number in the spinner is:

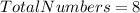
$TotalNumbers=8\text{ }$

a. By definition, Odds Numbers are those numbers that are not divisible by 2.

In this case, you can identify that the Odd Numbers the spinner has are:

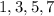
Knowing that there are four Odd Numbers, you can determine that:

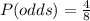
b. By definition, Even Numbers are divisible by 2.

In this case, you can identify that the Even Numbers in the spinner are:

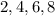
Knowing that there are four Even Numbers, you get:

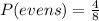
c. Since there is only one number 3:

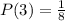
d. By definition:

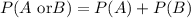
$P(A\text{ or}B)=P(A)+P(B)$

Then:

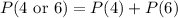
$P(4\text{ or }6)=P(4)+P(6)$

Since there is only one number 4 and only one number 6:

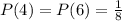
Therefore:

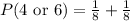
$P(4\text{ or }6)=(1)/(8)+(1)/(8)$

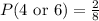
$P(4\text{ or }6)=(2)/(8)$

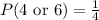
$P(4\text{ or }6)=(1)/(4)$

e. Since there is only one number 7:

f. Knowing that there is only one number 1:

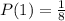
Hence, the answers are:

a.

b.

c.

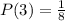
d.

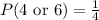
$P(4\text{ or }6)=(1)/(4)$

e.

f.